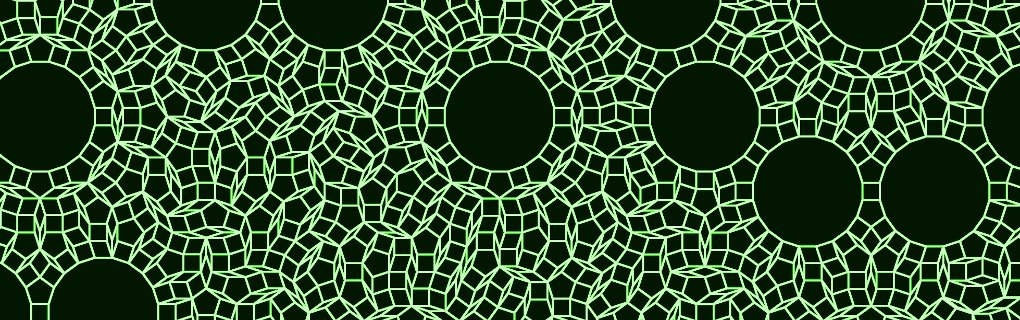
The example of the 5.5.10 tiling in the last section raises the question of whether other "forbidden" vertex figures can be extended to tilings using rhombs.
It turns out that they all can, resulting in this simple but beautiful result:
Vertex figure extension theorem
Any vertex figure of regular polygons can be extended to a periodic edge-to-edge tiling of the plane using a finite set of regular polygon or rhomb prototiles.
I don't claim that this is an original result. On the other hand, I have never seen it stated or proved anywhere before.
Given the results I have presented up to this point, all that is needed to complete the proof is to present periodic tilings including the 6 "forbidden" vertex types using rhomb and regular polygon prototiles. I will do this in the following pages. So far as I know, these tilings have never appeared anywhere on the Internet before and may be completely new.
A conjecture
It is a common situation in mathematics to discover that a problem within a system can only be solved by extending the system. Another example is the creation of the complex number system from the integers, first by adding fractions, next by adding non-fractional "irrational" numbers such as the square root of two, then by adding transcendental numbers such as π and finally by adding the "imaginary" number i, the square root of -1.
i is an especially interesting case - it was needed to establish the fundamental theorem of algebra, which guarantees that every non-trivial polynomial equation has at least one solution. It turns out that the fundamental theorem of algebra is still true even if the polynomial coefficients are allowed to be complex numbers. No further extension is needed. It is sometimes said that such systems are "complete".
The equivalent completeness criteria for tiling theory would be the following conjecture:
Vertex figure extension conjecture
Any vertex figure of regular polygons and rhombs can be extended to a periodic edge-to-edge tiling of the plane using a finite set of regular polygon and rhomb prototiles.
Although I am presenting this in the form of a conjecture, I won't speculate myself at this point whether it is true or false.
If this conjecture is true, it would be a very interesting result. We could not prove this conjecture using the method of enumerating vertex figures that we used for regular polygons, however, because there are an infinite number of vertex figures involving rhombs and regular polygons.
If the conjecture is false, then it raises the question of whether the set of prototiles could be extended still further beyond rhombs to create a "complete" set of prototiles. Disproving the conjecture would also raise the question of whether it might be possible to determine which vertex figures (including rhombs) can be extended to periodic tilings.
As is usual in mathematics, the solution of one problem opens the doors to many others ...