Kepler described the vertex types 3.3.3.3.3.3, 4.4.4.4 and 6.6.6 as the "most" perfect congruences. These are the only vertex figures with a single prototile.
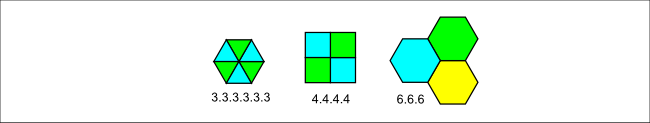
Each of these figures can be extended to a tiling of the plane that has a single prototile and a single vertex type. These three tilings are called the regular tilings and consist respectively of a single triangle, square or hexagon repeated indefinitely.
The tilings shown on this page are taken from Wikipedia (as shown in the article List of uniform tilings).
Translational units
The regular tilings (and most tilings described on this site) are periodic - they can be created by repeating the same finite patch of tiles indefinitely. All periodic tilings have translational units - finite patches of tiles that can be used to generate the tiling through translation alone without rotation or reflection. Any periodic tiling has an infinite number of possible translational units. I have chosen a reasonably simple one to exhibit (in the second column) for each tiling below.
Skeleton tilings
The external edges of a translational unit can be considered to be a polygon (usually irregular) that tiles the plane by itself. I have called the translational unit tiling the skeleton tiling on this site and provide this for each tiling (in the third column) below. The translational unit and the skeleton tiling together can be used to construct the original tiling and so give important information (indeed complete information) about its structure.
As you can see, a translational unit for the 3.3.3.3.3.3 tiling consists of two triangles. A single square and a single hexagon suffices for the 4.4.4.4 and 6.6.6 tilings respectively and so in these cases, the skeleton tiling is the same as the main tiling.
The translational units and skeleton tilings are quite simple for the regular tilings. They will become more complex as we consider other tilings later on this site.
List of regular tilings
3.3.3.3.3.3
 |
 |
 |
4.4.4.4
 |
 |
 |
6.6.6
 |
 |
 |